ETC Best Paper Award (cake debt)
In April 2021, myself and my coauthor Graham Pullan won the Best Paper Award at the 14th European Turbomachinery Conference for our paper on turbine acoustic impedance. This post is subtitled “cake debt”, as it is the Whittle Lab custom to bring in baked goods on such occasions, but presently I am still working from home.
This post summarises and explains the work that went into the paper, with a less-technical slant than the publication itself. First, I describe the engineering challenge of combustion instability in gas turbines, which is the motivation for research on this topic. I then say a bit about the numerical and analytical methods from the paper. Finally, I discuss our results and their implications for gas turbine design.
Physical principles of combustion instability
The relevant physics are nicely demonstrated by a Rijke tube,
Blowing over the neck of an empty bottle produces sound at a certain frequency. Smaller bottles produce higher notes, and vice-versa. Similarly, the air in a pipe will resonate at its natural frequency when given a suitable push. However, this alone does not explain the unusual behaviour of a Rijke tube.
The secret is that inside the tube, about a quarter of the way along, is a thick wire mesh. The mesh first stores heat from the flame, and subsequently transfers heat to the air as it cools down. Under the right conditions, there is a positive feedback loop between resonance and heating of the air: as the mesh cools it provides the push required to make noise.
Lord Rayleigh explained the mechanism in 1878, in a lecture at the Royal Institution which was later published in Nature. He likened the Rijke tube to an organ pipe, where the energy needed to maintain the vibration comes from heat stored in the mesh, instead of being provided by a pump as in a church.

Rayleigh understood the physical principles of combustion instability in 1878.
Rayleigh also described why sound is only produced in one orientation:
If heat be given to the air at the moment of greatest condensation, or taken from it at the moment of greatest rarefaction, the vibration is encouraged. On the other hand, if heat be given at the moment of greatest rarefaction, or abstracted at the moment of greatest condensation, the vibration is discouraged.
To put it another way: if the mesh adds heat when the air is at high pressure, sound is produced. Heat transfer from the mesh depends on the air velocity through it, which changes as the tube is rotated to different positions (hot air rises). The heat transfer only lines up with high pressure when the mesh is in the bottom half of the tube.
The Engineering challenge of combustion instability
With the dawn of the space race, combustion instability moved from a scientific curiosity to an intractable engineering problem. The effects of acoustic resonance forced by burning rocket fuel are much less benign than those forced by a hot wire mesh:

The aftermath of combustion instability in a rocket engine test, NASA.
As shown in the photo above from NASA Glenn, the development of rocket engines to power the Saturn V and moon landings was beset by problems. Engines would develop violent vibrations at particular frequencies, sometimes with explosive results, but it was not clear how the design of the engines could be changed to prevent this. The solution is much more complex than simply rotating the Rijke tube. NASA tested hundreds of engines, and eventually eliminated the problems by trial and error.
Gas turbines, used for power generation, also suffer from combustion instabilities. Our work with Mitsubishi Heavy Industries is to improve tools for predicting the stability of a proposed design before it is manufactured. With reliable predictions, much of the old trial and error can be avoided, although the final designs must still be thoroughly tested!
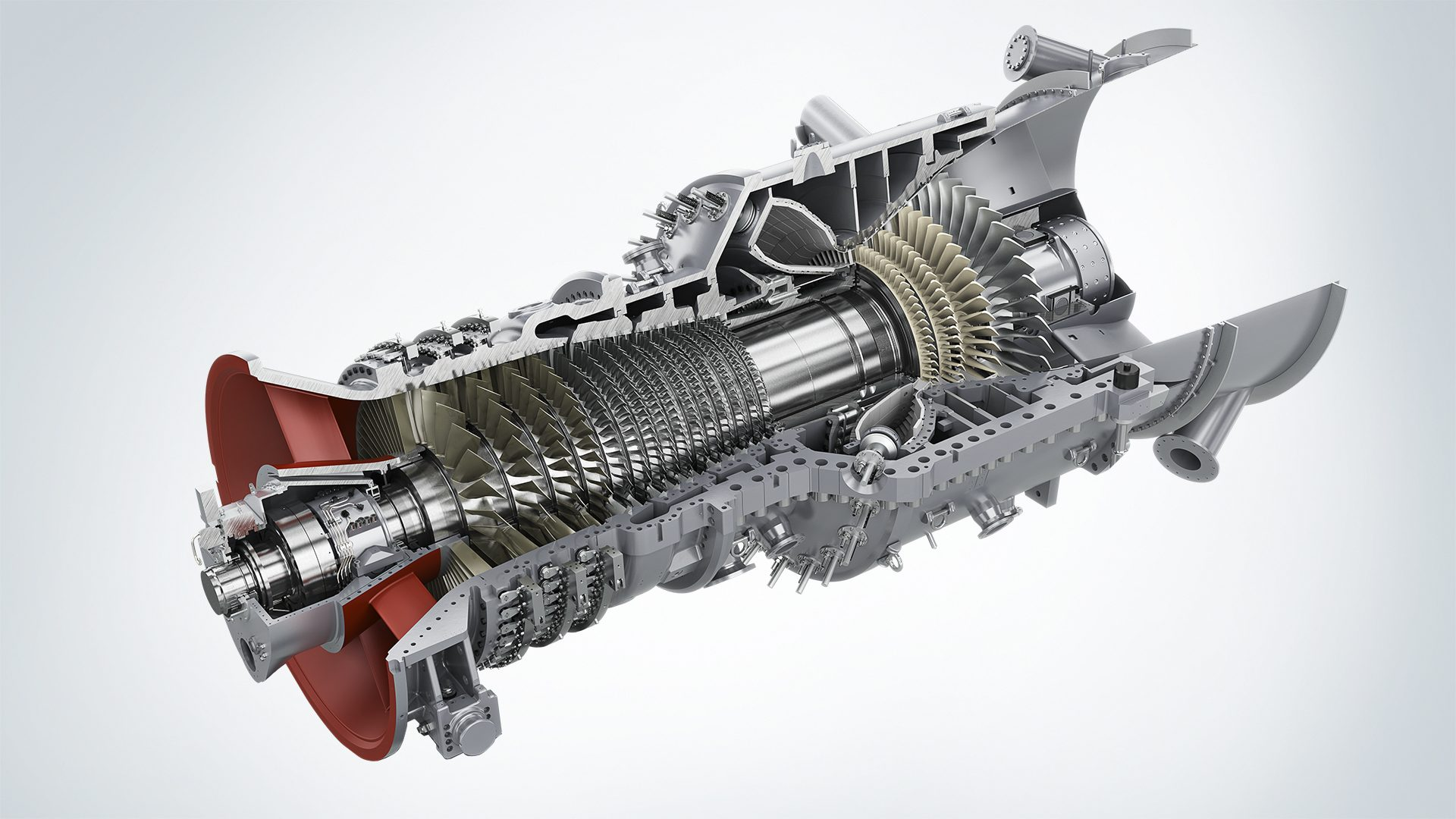
Cross section of a Siemens gas turbine, combustion chambers located near the center (image credit: Siemens website).
Turbine acoustic impedance
An important parameter which affects stability is the acoustic impedance of the turbine, which quantifies the strength of sound waves that are reflected from the turbine back towards the flame. This is related to how echoy the combustion chamber is:
The acoustic impedance will depend on the design of the turbine, and we developed a mathematical model to predict it for a given turbine.
Mathematical model
We start by making some simplifying approximations, one of the pillars of Engineering science. The turbine contains blades, or spinning things that extract power from the hot gas flowing past, a bit like a windmill. Although a turbine is a three-dimensional object, in our model we first squash it radially so it looks flat and two-dimensional. Then, we make the blades very skinny and close together so that the flow becomes one-dimensional, i.e. does not vary horizontally in the picture below.
Reducing the three-dimensional flow field to a simpler one-dimensional description.
We neglect viscosity and heat conduction effects. Practically speaking, this means that acoustic waves don’t lose any energy, and would propagate forever in an infinitely long duct.
Lastly, we consider small perturbations only, where the size of fluctuations in pressure are much less than the average value. This is true of most sound waves — the threshold of human hearing corresponds to a fractional perturbation less than one billionth of atmospheric pressure.
If we do all that, the flow field at any point is a sum of three contributions:
- A pressure wave moving upstream;
- A pressure wave moving downstream;
- A temperature hot-spot moving at the flow speed.
To calculate the impedance, we need to know the amplitudes of each of these waves, which we get by solving a system of simultaneous linear equations (GCSE-level maths!). Now we have simplified the problem, life is much easier.
Computational fluid dynamics simulations
Having a neat mathematical model is great, but nobody believes your results unless you have proof you get the right answers. Ideally, we would compare the predictions with measurements of acoustic impedance on actual turbines. This presents a bit of a problem for us — building and running an entire gas turbine is ruinously expensive, and most measurement equipment doesn’t last long at 1600K. You would also need a massive stack of speakers to generate the sound waves required to set up the test.
So to get around this, we ran some detailed computations of the flow in gas turbines, removing the assumptions of the mathematical model. ‘Measuring’ things in a computer simulation is much easier: in fact, we have full knowledge of every point in the flow field. These simulations make some pretty pictures:
Animation of soundwaves reflecting from a turbine stage using computational fluid dynamics.
Now comes the moment of truth, where we compare the simple model and detailed simulation in the figure below. On the vertical axis is acoustic impedance (reflectiveness or amount of echo), and on the horizontal axis is frequency (the pitch of the sound). Fortunately, we have a close match between the crosses and the lines, and we can move on to using the model for interesting stuff.
Turbine acoustic impedance predicted by mathematical model and detailed simulations (CFD). There is an encouraging agreement between the two approaches.
Variations in impedance around the turbine design space
Now we have a simple and quick mathematical model, we can look at hundreds of turbine designs in just a few minutes on a laptop computer. Back in 1960s, this would have taken NASA many months at great expense. We use this capability to look at how changes to the turbine design affect acoustic impedance.
It turns out that the most important parameter is how much turning occurs in the first row of blades. When the turning is high, the blades act as blockers to the sound wave and increase the acoustic impedance.
The mathematical model shows that turbines with more turning through the first blade row have a higher acoustic impedance, due to geometric blockage.
Other parameters affect the impedance by changing the area ratio through the turbine. If we try to force a sound wave through a small gap, it will be reflected back towards us; in contrast brass musical instruments have pipes which increase in area to trasmit as much sound as possible through them.
We also found that Mach number, the ratio of gas speed to speed of sound, has a strong effect on the acoustic impedance: higher Mach numbers make the turbine more reflective. For the interested reader, there is much more detail on the turbine design study in the full paper.
Summary
Combustion instability, when there is a positive feedback loop between acoustic resonance and heating, is a major problem for gas turbines. The stability of a combustion system depends on the acoustic impedance, or how echoy the combustion chamber is. In our paper, we developed a mathematical model for the acoustic impedance of a turbine by making some approximations. Then, we proved it was correct by comparing to full calculations of the flow field. Lastly, we used the model to explore the turbine design space, and found that the acoustic impedance is most strongly influenced by turning through the first turbine blade row.